Difference between revisions of "Carbon nanotube"
ChristiaanK (talk | contribs) m (See also) |
(Added use as a greenhouse nano-particle and a link.) |
||
| (3 intermediate revisions by 2 users not shown) | |||
| Line 4: | Line 4: | ||
[[File:Carbon_nanotube_from_graphene.png|frame|right|Figure 1]] | [[File:Carbon_nanotube_from_graphene.png|frame|right|Figure 1]] | ||
Carbon nanotubes are classified according to their concentricity, tube thickness and chirality (or zigzag/armchair status, if not chiral). There are also a number of interesting nanotube-derived materials.<br /> | Carbon nanotubes are classified according to their concentricity, tube thickness and chirality (or zigzag/armchair status, if not chiral). There are also a number of interesting nanotube-derived materials.<br /> | ||
| − | Figure 1 is a graphical depiction of how carbon nanotubes are equivalent to rolled-up graphene. In each of the colours, the solid line (overlapped by the dotted line for yellow) depicts a perpendicular cross-section of the nanotube. (This means that the axis of the tube runs at a right angle to that line.) The two dotted line indicates how the characteristic vector (n, m) of the nanotube is calculated. The tesselation wraps around so that the hexagon centred on O takes the place of the hexagons centred on A to D, respectively. The angle theta indicates how strongly the nanotube spirals, but not whether it is left-handed or right-handed (if chiral) nor how thick it is. | + | Figure 1 is a graphical depiction of how carbon nanotubes are equivalent to rolled-up graphene. In each of the colours, the solid line (overlapped by the dotted line for yellow) depicts a perpendicular cross-section of the nanotube. (This means that the axis of the tube runs at a right angle to that line.) The two dotted line indicates how the characteristic vector (n, m) of the nanotube is calculated. n is the number of hexagons the horizontal line (which corresponds to a zigzag nanotube) cuts through and m is the number of hexagons that the second dotted line cuts through. (n,m) is (2,2) for A, (4,2) for B, (3,3) for C and (4,0) for D. The tesselation wraps around so that the hexagon centred on O takes the place of the hexagons centred on A to D, respectively. The angle theta indicates how strongly the nanotube spirals, but not whether it is left-handed or right-handed (if chiral) nor how thick it is. |
===Layering=== | ===Layering=== | ||
'''Multi-walled carbon nanotubes''' (MWNT) consist of more than one tube of different sizes inside one another. Single tubes are known as '''single-walled carbon nanotubes''' (SWNT).<ref name=Housecroft_Sharpe /><br/> | '''Multi-walled carbon nanotubes''' (MWNT) consist of more than one tube of different sizes inside one another. Single tubes are known as '''single-walled carbon nanotubes''' (SWNT).<ref name=Housecroft_Sharpe /><br/> | ||
===Chirality=== | ===Chirality=== | ||
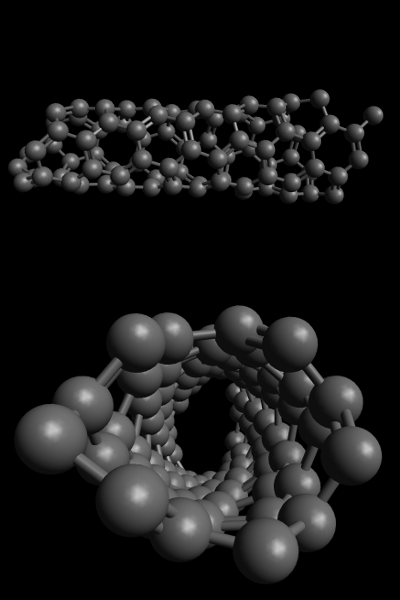
| + | [[File:SWNT_4_2_LH.png|frame|left|Figure 2]] | ||
The shape of a carbon nanotube can be described using the value <math>\theta</math>, the (absolute value of the) angle at which it is twisted (relative to a zigzag nanotube) when this tube is conceptually made from a sheet of graphite. | The shape of a carbon nanotube can be described using the value <math>\theta</math>, the (absolute value of the) angle at which it is twisted (relative to a zigzag nanotube) when this tube is conceptually made from a sheet of graphite. | ||
*<math>\theta = 0^\circ</math> for '''zigzag nanotubes''', which may be metallic conductors or semiconductors. A cross-section of a zigzag carbon nanotube forms a tidy zigzag pattern. | *<math>\theta = 0^\circ</math> for '''zigzag nanotubes''', which may be metallic conductors or semiconductors. A cross-section of a zigzag carbon nanotube forms a tidy zigzag pattern. | ||
*<math>\theta = 30^\circ</math> for '''armchair''' nanotubes, which are [[metal|metallic conductors]]. A cross-section of an armchair carbon nanotube forms a stepped pattern. | *<math>\theta = 30^\circ</math> for '''armchair''' nanotubes, which are [[metal|metallic conductors]]. A cross-section of an armchair carbon nanotube forms a stepped pattern. | ||
*<math>0^\circ < \theta < 30^\circ</math> for all other nanotubes, which are called [[chirality|chiral]] carbon nanotubes. They may be either left-handed (following the adjacent hexagons in a clockwise direction leads you to spiral upwards along the tube) or right-handed (following the adjacednt hexagons in an anticlockwise direction leads you to spiral upwards along the tube). Chiral nanotubes may be metallic conductors or semiconductors.<br /> | *<math>0^\circ < \theta < 30^\circ</math> for all other nanotubes, which are called [[chirality|chiral]] carbon nanotubes. They may be either left-handed (following the adjacent hexagons in a clockwise direction leads you to spiral upwards along the tube) or right-handed (following the adjacednt hexagons in an anticlockwise direction leads you to spiral upwards along the tube). Chiral nanotubes may be metallic conductors or semiconductors.<br /> | ||
| − | To more fully describe a carbon nanotube, the value <math>C_h = na_1 + ma_2</math> can be used. The values of <math>a_1</math> and <math>a_2</math> are the widths of the hexagons which make up the nanotube, measured respectively in the directions in which n and m are measured (see Figure 1). <math>C_h = na_1 + ma_2</math> is the Manhattan distance for one full revolution around the tube. For zigzag nanotubes, <math>m=0</math> and for armchair nanotubes, <math>m=n</math>. | + | To more fully describe a carbon nanotube, the value <math>C_h = na_1 + ma_2</math> can be used. The values of <math>a_1</math> and <math>a_2</math> are the widths of the hexagons which make up the nanotube, measured respectively in the directions in which n and m are measured (see Figure 1). <math>C_h = na_1 + ma_2</math> is the Manhattan distance for one full revolution around the tube. For zigzag nanotubes, <math>m=0</math> and for armchair nanotubes, <math>m=n</math>.<br /> |
| + | Figure 2 illustrates a left-handed SWNT (4,2). Note that if you were to complete the partially cut-off hexagon on the right of the side-view and then continue with those that follow, you would spiral to the right according to the [[chirality|left-hand rule]]. | ||
===Modified carbon nanotubes=== | ===Modified carbon nanotubes=== | ||
| Line 21: | Line 23: | ||
Commercial production of carbon nanotubes takes place by [[chemical vapour deposition]], laser vaporisation (of [[graphite]]) or electrical arcing between graphite electrodes<ref name=Housecroft_Sharpe />. The electric arc method tends to create MWNTs. Lengths produced are currently in the micrometre range, while thicknesses vary from about 1nm for the thinnest SWNTs and 100nm for the thickest MWNTs<ref name=Housecroft_Sharpe /> | Commercial production of carbon nanotubes takes place by [[chemical vapour deposition]], laser vaporisation (of [[graphite]]) or electrical arcing between graphite electrodes<ref name=Housecroft_Sharpe />. The electric arc method tends to create MWNTs. Lengths produced are currently in the micrometre range, while thicknesses vary from about 1nm for the thinnest SWNTs and 100nm for the thickest MWNTs<ref name=Housecroft_Sharpe /> | ||
| − | ==Possible uses | + | ==Possible uses on Mars== |
*Carbon nanotubes are perhaps most notable for their extreme tensile strength, making them an ideal material for spacecraft construction. | *Carbon nanotubes are perhaps most notable for their extreme tensile strength, making them an ideal material for spacecraft construction. | ||
*They are also one of the only materials which have a high enough tensile strength that they might, possibly, enable a [[space elevator]] on earth. | *They are also one of the only materials which have a high enough tensile strength that they might, possibly, enable a [[space elevator]] on earth. | ||
*The electrical properties of carbon nanotubes suggest that they might some day be used in computers. | *The electrical properties of carbon nanotubes suggest that they might some day be used in computers. | ||
*Excellent resistance to thermal shock and high temperatures (which is shared by all forms of graphite, graphene, carbon nanotubes and carbon fibre), in combination with high tensile strength, raises the question of whether hypersonic parachute designs might improve the usefulness of [[aerobraking]]. | *Excellent resistance to thermal shock and high temperatures (which is shared by all forms of graphite, graphene, carbon nanotubes and carbon fibre), in combination with high tensile strength, raises the question of whether hypersonic parachute designs might improve the usefulness of [[aerobraking]]. | ||
| + | *It is possible that a carbon nanotube would make an excellent [[Greenhouse nano-particles]], for warming the planet, but this has not yet been proven. | ||
==See also== | ==See also== | ||
| Line 39: | Line 42: | ||
<references /> | <references /> | ||
| − | [[Category: | + | [[Category:Materials]] |
Latest revision as of 23:32, 13 September 2024
A carbon nanotube is one or more concentric sheets of graphene rolled into tubes. They are amongst the stiffest and strongest materials known to mankind and their electrical properties can be varied from metallic to semiconducting.[1]
Contents
Types
Carbon nanotubes are classified according to their concentricity, tube thickness and chirality (or zigzag/armchair status, if not chiral). There are also a number of interesting nanotube-derived materials.
Figure 1 is a graphical depiction of how carbon nanotubes are equivalent to rolled-up graphene. In each of the colours, the solid line (overlapped by the dotted line for yellow) depicts a perpendicular cross-section of the nanotube. (This means that the axis of the tube runs at a right angle to that line.) The two dotted line indicates how the characteristic vector (n, m) of the nanotube is calculated. n is the number of hexagons the horizontal line (which corresponds to a zigzag nanotube) cuts through and m is the number of hexagons that the second dotted line cuts through. (n,m) is (2,2) for A, (4,2) for B, (3,3) for C and (4,0) for D. The tesselation wraps around so that the hexagon centred on O takes the place of the hexagons centred on A to D, respectively. The angle theta indicates how strongly the nanotube spirals, but not whether it is left-handed or right-handed (if chiral) nor how thick it is.
Layering
Multi-walled carbon nanotubes (MWNT) consist of more than one tube of different sizes inside one another. Single tubes are known as single-walled carbon nanotubes (SWNT).[1]
Chirality
The shape of a carbon nanotube can be described using the value , the (absolute value of the) angle at which it is twisted (relative to a zigzag nanotube) when this tube is conceptually made from a sheet of graphite.
- for zigzag nanotubes, which may be metallic conductors or semiconductors. A cross-section of a zigzag carbon nanotube forms a tidy zigzag pattern.
- for armchair nanotubes, which are metallic conductors. A cross-section of an armchair carbon nanotube forms a stepped pattern.
- for all other nanotubes, which are called chiral carbon nanotubes. They may be either left-handed (following the adjacent hexagons in a clockwise direction leads you to spiral upwards along the tube) or right-handed (following the adjacednt hexagons in an anticlockwise direction leads you to spiral upwards along the tube). Chiral nanotubes may be metallic conductors or semiconductors.
To more fully describe a carbon nanotube, the value can be used. The values of and are the widths of the hexagons which make up the nanotube, measured respectively in the directions in which n and m are measured (see Figure 1). is the Manhattan distance for one full revolution around the tube. For zigzag nanotubes, and for armchair nanotubes, .
Figure 2 illustrates a left-handed SWNT (4,2). Note that if you were to complete the partially cut-off hexagon on the right of the side-view and then continue with those that follow, you would spiral to the right according to the left-hand rule.
Modified carbon nanotubes
It is sometimes desirable to modify carbon nanotubes to make them soluble in either water or organic solvents, bond them to another material or perform some other function.
One of the ways to achieve this is to react the carbon nanotubes with chemicals, such as fluorine, which can bond to their surface. Since each carbon atom has a double bond with one of its neigbours, it is possible to bond carbon nanotubes to other molecules or elements without disrupting the physical structure. However, this reduces electrical conductivity.[1].
Production
Commercial production of carbon nanotubes takes place by chemical vapour deposition, laser vaporisation (of graphite) or electrical arcing between graphite electrodes[1]. The electric arc method tends to create MWNTs. Lengths produced are currently in the micrometre range, while thicknesses vary from about 1nm for the thinnest SWNTs and 100nm for the thickest MWNTs[1]
Possible uses on Mars
- Carbon nanotubes are perhaps most notable for their extreme tensile strength, making them an ideal material for spacecraft construction.
- They are also one of the only materials which have a high enough tensile strength that they might, possibly, enable a space elevator on earth.
- The electrical properties of carbon nanotubes suggest that they might some day be used in computers.
- Excellent resistance to thermal shock and high temperatures (which is shared by all forms of graphite, graphene, carbon nanotubes and carbon fibre), in combination with high tensile strength, raises the question of whether hypersonic parachute designs might improve the usefulness of aerobraking.
- It is possible that a carbon nanotube would make an excellent Greenhouse nano-particles, for warming the planet, but this has not yet been proven.