Difference between revisions of "Mass driver"
(Did math to find the lengths of mass drivers. Olympus Mons is indeed large enough to place a mass driver on it. Several other edits.) |
(Did some work on heating of mass driver launch. sources are a bunch of google searches.) |
||
| Line 23: | Line 23: | ||
[[Image:MassDriverOnOlympusMons.png|thumb|right|300px|Using the shape of Olympus Mons]] | [[Image:MassDriverOnOlympusMons.png|thumb|right|300px|Using the shape of Olympus Mons]] | ||
| − | Since the viability of a mass driver increases with a lowered atmospheric pressure, the location of the mass driver's muzzle should be as high as possible. The top (or rim) of [[Olympus Mons]] (the highest mountain in the [[solar system]]) might be a good choice. It is about 625 km wide and 25 km tall. It has an air pressure of about 40 Pa at top.<ref name="stanford">[http://www-star.stanford.edu/projects/mgs/tps-public.html Public Access to Standard Temperature-Pressure Profiles] Standard Pressure Profiles measured by MGS Radio Science team at 27 km range from approx 30 to 50 pascals</ref><ref name="stanlate">[http://www-star.stanford.edu/projects/mgs/late.html Late Martian Weather!] ''stanford.edu'' temperature/pressure profiles 1998 to 2005</ref><!-- verifier: assuming 27km elevation, select various datapoints, subtract surface elevation from 27km and read off pressure from graph; errors around ±5% --> | + | Since the viability of a mass driver increases with a lowered atmospheric pressure, the location of the mass driver's muzzle should be as high as possible. The top (or rim) of [[Olympus Mons]] (the highest mountain in the [[solar system]]) might be a good choice. It is about 625 km wide and 25 km tall. It has an air pressure of about 40 Pa at top.<ref name="stanford">[http://www-star.stanford.edu/projects/mgs/tps-public.html Public Access to Standard Temperature-Pressure Profiles] Standard Pressure Profiles measured by MGS Radio Science team at 27 km range from approx 30 to 50 pascals</ref><ref name="stanlate">[http://www-star.stanford.edu/projects/mgs/late.html Late Martian Weather!] ''stanford.edu'' temperature/pressure profiles 1998 to 2005</ref><!-- verifier: assuming 27km elevation, select various datapoints, subtract surface elevation from 27km and read off pressure from graph; errors around ±5% --> (Note that 40 Pa = 0.0004 bar, so this is a high grade vacuum.) |
| + | |||
| + | Olympus Mon also offers a natural ramp. The mass driver must be almost perfectly straight (there is a slight curve for coriollis forces caused by the rotation of the planet while launching) so tunnels, cuts and bridges will have to be made. | ||
Pavonus Mons is a tall volcano directly on the Equator which could be suitable if equatorial launches are preferred. It is 375 km wide and 14 km high, so an acceleration of 9 gees for 57 seconds would require a mass driver 143 km long. | Pavonus Mons is a tall volcano directly on the Equator which could be suitable if equatorial launches are preferred. It is 375 km wide and 14 km high, so an acceleration of 9 gees for 57 seconds would require a mass driver 143 km long. | ||
| Line 29: | Line 31: | ||
==Open issues== | ==Open issues== | ||
| − | *How strong is the effect of air friction at an altitude of the Olympus Mons top? Does it have a damaging effect on a steel hull? | + | *How strong is the effect of air friction at an altitude of the Olympus Mons top? Does it have a damaging effect on a steel hull? This is mach 14.5 which is about 1/5 of the atmospheric heating caused by reentry on Earth IF the air pressure was equal. However, maximum heating in Earth reentry is at about 100km attitude, and 40 Pa is 1/4 this. So steel should be able to handle this amount of heating, especially since it would rapidly decrease as the ship went higher. The friction WOULD be enough that the minimum speed of 5,020 m/s would not reach orbit. Either the mass driver must give extra speed to make up for friction, or the payload would want a small rocket to over come the velocity lost, and to circularize the orbit once high enough. |
| − | *Normal people can withstand 9 gravities (gees) of acceleration for about 1 minute. 6 gees of acceleration can be withstood for 10 | + | *Normal people can withstand 9 gravities (gees) of acceleration for about 1 minute. 6 gees of acceleration can be withstood for around 10 minutes. |
==references== | ==references== | ||
Revision as of 19:20, 11 March 2021
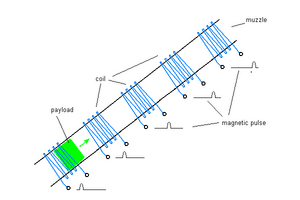
The transport from the surface of Mars to Mars' orbit and vice versa can be achieved by a mass driver or electromagnetic catapult. The idea is to install an electrical linear motor on the surface, pointing towards the desired orbit in an appropriate angle.
A mass driver is powered electrical and requires no chemical fuels. While on Earth this would not work for lifting people to orbit, the conditions of Mars might allow it. The acceleration of persons is limited to a few g, so the linear motor must be very long for that case.
Sky ramps are very similar, but they use rockets rather than a mass driver, and only acts as a reusable first stage rather than launching a ship directly into orbit.
Launching a payload
The payload is accelerated along the linear motor. Until it reaches the end of the linear motor it gains enough velocity to get to the desired orbit just by inertia. The velocity must be higher than the escape velocity because of the braking effect of the Martian atmosphere.
Mars' escape velocity is 5,020 m/s. If you accelerated at 6 gravities, then this would take ~86 seconds to reach orbital velocity. This would require a mass driver which would be 218 km long. (This distance is about 1/3 of the of the width of Olympus Mons. If you wished to use a smaller volcano, then a higher acceleration would be needed.)
The payload would need to have small rockets to circularize the orbit once on the other side of the planet.
Landing a payload
Just like launching, the landing of a payload can be done. The trajectory of the payload from outer space or from an orbit to the muzzle of the mass driver must be adjusted with nearly perfect accuracy. When entering the muzzle, the payload has a very high velocity, nearly as high as the escape velocity. The payload is decelerated by the linear motor until it comes to a halt.
Possible locations
Since the viability of a mass driver increases with a lowered atmospheric pressure, the location of the mass driver's muzzle should be as high as possible. The top (or rim) of Olympus Mons (the highest mountain in the solar system) might be a good choice. It is about 625 km wide and 25 km tall. It has an air pressure of about 40 Pa at top.[1][2] (Note that 40 Pa = 0.0004 bar, so this is a high grade vacuum.)
Olympus Mon also offers a natural ramp. The mass driver must be almost perfectly straight (there is a slight curve for coriollis forces caused by the rotation of the planet while launching) so tunnels, cuts and bridges will have to be made.
Pavonus Mons is a tall volcano directly on the Equator which could be suitable if equatorial launches are preferred. It is 375 km wide and 14 km high, so an acceleration of 9 gees for 57 seconds would require a mass driver 143 km long.
Open issues
- How strong is the effect of air friction at an altitude of the Olympus Mons top? Does it have a damaging effect on a steel hull? This is mach 14.5 which is about 1/5 of the atmospheric heating caused by reentry on Earth IF the air pressure was equal. However, maximum heating in Earth reentry is at about 100km attitude, and 40 Pa is 1/4 this. So steel should be able to handle this amount of heating, especially since it would rapidly decrease as the ship went higher. The friction WOULD be enough that the minimum speed of 5,020 m/s would not reach orbit. Either the mass driver must give extra speed to make up for friction, or the payload would want a small rocket to over come the velocity lost, and to circularize the orbit once high enough.
- Normal people can withstand 9 gravities (gees) of acceleration for about 1 minute. 6 gees of acceleration can be withstood for around 10 minutes.
references
- ↑ Public Access to Standard Temperature-Pressure Profiles Standard Pressure Profiles measured by MGS Radio Science team at 27 km range from approx 30 to 50 pascals
- ↑ Late Martian Weather! stanford.edu temperature/pressure profiles 1998 to 2005